In this module you investigated the following questions:

• How can trigonometry be used to model and solve problems involving motion?
• What are the uses and limitations of a trigonometric identity?

In this module you applied trigonometry to a variety of different situations involving motion. In Lesson 1 you used the tangent function to model the motion of cars in a straight line. In Lesson 2 you used different trigonometric functions to model predictable, cyclical motion, like the motion of pistons or tides. In Lessons 3, 4, and 5 you learned how to verify, simplify, and prove trigonometric identities. You then used these identities to simplify expressions and solve equations.

 

You applied your understanding of using trigonometric functions to model and solve problems in the Module 5 Project: Pushing the Limits of Vehicle Performance. In this project you used trigonometry to determine the distances, speeds, and angles required to jump vehicles over large obstacles. You modelled the speed of different gears and determined the speed of a vehicle. You also proved that the distance travelled by a motorcycle leaving a jump can be expressed by a trigonometric equation. You then solved that equation to determine a safe launch angle.

 

Following are some of the key ideas that you learned in each lesson.

 

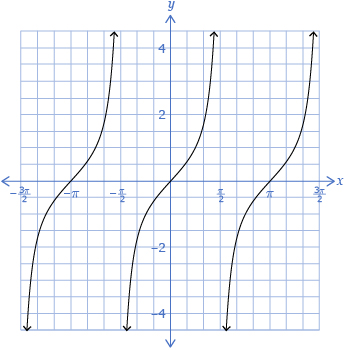
Lesson 1	y = tan x is a periodic function that has regular asymptotes and a range of all real numbers.
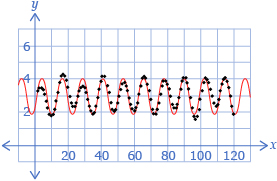
Lesson 2	Some scenarios can be modelled using trigonometric functions. Care must be taken when deciding when a model is reasonable and how it should be applied.
Lesson 3	An identity is an equation that is true for any value of the variable that is permissible on both sides of the equation. Identities can be verified numerically or graphically.
Lesson 4	A trigonometric identity can be used to simplify expressions and solve trigonometric equations.
Lesson 5	Proving a trigonometric identity shows how you know for certain the identity is true for all permissible values.